A novel ADRC-based design for a kind of flexible aerocraft

Abstract

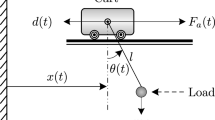
The paper presents a novel control design, which is based on the idea of active disturbance rejection control (ADRC), for a kind of flexible aerocraft whose controlled variable cannot be measured directly. Since the original frame of ADRC cannot be directly applied, the paper puts forward a new extended state observer (ESO) and the corresponding ADRC law. In order to assign the poles of the closed-loop system to ideal positions such that the vibration can be quickly suppressed, an elastic damping term is added into feedback law. The advantages of the new ESO for effectively estimating both the rigid mode and elastic mode from the measurements are discussed. Moreover, the analysis on the stability, the relative stability and the steady state of the closed-loop system is given. Finally, the effectiveness and robustness of the proposed ADRC are verified by simulations.

Appendix

Proof of Lemma 1

Since the pair $({\bar{A}},{\bar{C}}_1)$ is observable, the matrix $M_O$ is nonsingular. Denote

$$\begin{aligned} v(t) = M_O (X(t) - z(t) - M(t)), \end{aligned}$$

(a1)

the dynamics of v(t) is obtained as follows:

$$\begin{aligned} {\dot{v}}(t) = ({\bar{A}}_{e}-L_{e}{\bar{c}}_{e}) {v}(t) + H f_v, \end{aligned}$$

(a2)

where $L_{e}=M_O L,$ ${\bar{c}}_{e}={\bar{C}}_1 M_O^{-1},$

$$\begin{aligned} {\bar{A}}_{e} =M_O {\bar{A}} M_O^{-1}= \left[ \begin{array}{c@{~~~}c@{~~~}c@{~~}cc} 0&{}1&{}0 &{}0 &{} 0 \\ 0&{}0&{}1 &{}0 &{} 0 \\ 0&{}0&{}0 &{}1 &{} 0 \\ 0&{}0&{}0 &{}0 &{} 1\\ 0 &{} 0 &{} 0&{}-{\bar{\omega }}_1^2 &{} -2\xi {\bar{\omega }}_1 \end{array}\right] , \end{aligned}$$

(a3)

and

$$\begin{aligned} f_v = {\bar{c}}_{e} {\bar{A}}_{\mathrm{e}}^{5} M(t) +\textstyle \sum \limits _{j=1}^{3} C_1 A^{4-j} B_2 f^{(j)}+\textstyle \sum \limits _{j=1}^{3} C_1 A^{4-j} B_1 f_q^{(j)}. \end{aligned}$$

(a4)

Due to the assumption that the disturbance $f_q \in \mathbb{R}$ is bounded, $f\in \mathbb{R}$ and $f_q \in \mathbb{R}$ have the bounded first 3rd-order derivatives; $f_v$ is bounded.

Since the pair $({\bar{A}}_{e},~{\bar{c}}_{e})$ is observable, there exist ${\bar{L}}_{e,1}$, $\bar{L}_{e,2}$ and the invertible matrices $P_{L1}$ and ${P}_{L2}$ such that

$$\left\{\begin{aligned} & {\bar{A}}_{e}-{\bar{L}}_{e,1}{\bar{c}}_{e}= \beta P_{L1}^{-1} M_\varLambda P_{L1}, \\ &{\bar{A}}_{e}-\bar{L}_{e,2} {\bar{c}}_{e}= {P}_{L2}^{-1} M_\varLambda {P}_{L2}, \end{aligned}\right.$$

(a5)

where

$$\begin{aligned} M_\varLambda = \begin{bmatrix} {\lambda }_{1}&{} 0 &{} \cdots &{}0\\ 0&{}{\lambda }_{2}&{}\cdots &{} 0 \\ \vdots &{}\vdots &{} &{}\vdots \\ 0&{} 0 &{}\cdots &{}{\lambda }_{{5}} \end{bmatrix}. \end{aligned}$$

(a6)

The relationship between $P_{L1}$ and ${P}_{L2}$ is obtained as follows:

$$\begin{aligned} P_{L1}= {P}_{L2} T_P, \end{aligned}$$

(a7)

where $T_P = M_P^{-1}M_\beta M_P$ and

$$\begin{aligned} M_P=\begin{bmatrix} 1&{}0&{} 0&{}0 &{}0\\ 2\xi {\bar{\omega }}_1&{} 1&{}0&{}0 &{}0 \\ {\bar{\omega }}_1^2&{}2\xi {\bar{\omega }}_1&{} 1&{}0&{}0\\ 0&{}{\bar{\omega }}_1^2&{}2\xi {\bar{\omega }}_1&{} 1&{}0\\ a&{}0&{}{\bar{\omega }}_1^2&{}2\xi {\bar{\omega }}_1&{} 1 \end{bmatrix}, ~~M_{\beta } = \begin{bmatrix} \beta ^{4} &{}0&{} \cdots &{}0\\ 0&{}\beta ^{3}&{}\cdots &{}0\\ \vdots &{}\vdots &{} &{}\vdots \\ 0&{}0&{}\cdots &{}1 \end{bmatrix}. \end{aligned}$$

(a8)

a is a constant. Denote $\tilde{v}(t) = T_P v(t)$, from (a2), (a5) and (a7), there is

$$\begin{aligned} \dot{\tilde{v}}(t) = \beta ({\bar{A}}_{e}-\bar{L}_{e,2}{\bar{c}}_{e}){\tilde{v}}(t) - H f_v. \end{aligned}$$

(a9)

Since the matrix $({\bar{A}}_{e}-\bar{L}_{e,2}{\bar{c}}_{e})$ is Hurwitz, there exists a positive definite matrix $Q_L$ such that

$$\begin{aligned} ({\bar{A}}_{e}-\bar{L}_{e,2}{\bar{c}}_{e})^{\mathrm{T}} Q_L+Q_L({\bar{A}}_{e}-\bar{L}_{e,2}{\bar{c}}_{e}) =-I. \end{aligned}$$

(a10)

Consider the Lyapunov function ${V}(t)={\tilde{v}}(t)^{\mathrm{T}} Q_L {\tilde{v}}(t)$. Then, we have

$$\begin{aligned} \lambda _{\min }(Q_L) \left\| {\tilde{v}}(t) \right\| ^2 \leqslant {V}(t) \leqslant \lambda _{\max }(Q_L) \left\| {\tilde{v}}(t) \right\| ^2 \end{aligned}$$

(a11)

and

$$\begin{aligned} \dfrac{{\rm d} \sqrt{ {V}(t)}}{{\rm d}t}&= \dfrac{ -\beta \left\| {\tilde{v}}(t) \right\| ^2-2 {\tilde{v}}(t)^{\mathrm{T}} Q_L H f_v}{2 \sqrt{ {V}(t)}}\nonumber \\&\leqslant -\dfrac{\beta }{2\lambda _{\max }(Q_L)} \sqrt{{V}(t)}+ \dfrac{\Vert Q_L \Vert | f_v |}{\sqrt{\lambda _{\min }(Q_L)}}. \end{aligned}$$

(a12)

Since $f_v$ is bounded, i.e., $|f_v |^2<\eta _{F}$ for some positive $\eta _{F}$, with the help of Gronwall lemma, it is indicated from (a12) that

$$\begin{aligned} \sqrt{{V}(t)} \leqslant \mathrm{e}^{-\frac{\beta t}{2\lambda _{\max }(Q_L)} } \sqrt{ {V}(0)} + \dfrac{2 \Vert Q_L\Vert \eta _{F} \lambda _{\max }(Q_L)}{\beta \sqrt{\lambda _{\min }(Q_L)}}. \end{aligned}$$

(a13)

Due to (a11), (a13) and

$$\begin{aligned} \Vert X(t)-z(t) - M(t)\Vert \leqslant \Vert T_Q^{-1} \Vert \Vert T_P^{-1}\Vert \Vert \tilde{v}(t)\Vert , \end{aligned}$$

(a14)

(15) is obtained. $\square$

Proof of Theorem 1

First, it will be proved that there exist the controller parameters $k_p,$ $k_d,$ $k_q$ and the observer parameter L, such that the closed-loop system is stable despite the uncertainties given in Table 1. If the controller parameters are chosen as $\omega _c=2,$ $\xi _c=2,$ $\omega _q=3,$ $\lambda (\bar{A}-L\bar{C})=10\{-0.9,-1,-0.95,-1.05,-1\},$ then it can be proved that the characteristic roots of the closed-loop system lie in the following regions, as shown in Table a1, i.e., the closed-loop system is stable, despite various uncertainties of the system parameters in the scopes of Table 1.

Table a1 Ranges of the characteristic roots

Since the closed-loop system is stable, the disturbances f and $f_q$ are bounded. Denote the transfer function from the total disturbance f to the estimation $z_i$ as $\dfrac{D_{fi}(s)}{N(s)}$ and the transfer function from the disturbance $f_q$ to the estimation $z_i$ as $\dfrac{D_{f_{qi}}(s)}{N(s)},$ $i=1,\ldots ,5.$ It can be obtained that

$$\begin{aligned} \left\{ \begin{array}{l} Z_1(s)=\Delta \varPhi (s)+\dfrac{D_{f1}(s)}{N(s)}F(s)+\dfrac{D_{f_{q1}}(s)}{N(s)}F_q(s),\\ D_{f1}(0)=0,~~\dfrac{D_{f_{q1}}(0)}{N(0)} =\dfrac{c_1}{{\bar{\omega }}_1^2},\\ Z_2(s)=Q_1(s)+\dfrac{D_{f2}(s)}{N(s)}F(s)+\dfrac{D_{f_{q2}}(s)}{N(s)}F_q(s),\\ D_{f2}(0)=0,~~\dfrac{D_{f_{q2}}(0)}{N(0)} =\dfrac{1}{{\bar{\omega }}_1^2},\\ Z_3(s)=s \Delta \varPhi (s)+\dfrac{D_{f3}(s)}{N(s)}F(s)+\dfrac{D_{f_{q3}}(s)}{N(s)}F_q(s),\\ D_{f3}(0)=0,~~{D_{f_{q3}}(0)} =0,\\ Z_4(s)=s Q_1(s)+\dfrac{D_{f4}(s)}{N(s)}F(s)+\dfrac{D_{f_{q4}}(s)}{N(s)}F_q(s),\\ D_{f4}(0)=0,~~{D_{f_{q4}}(0)} =0,\\ Z_5(s)=\dfrac{D_{f5}(s)}{N(s)}F(s)+\dfrac{D_{f_{q5}}(s)}{N(s)}F_q(s),\\ \dfrac{D_{f5}(0)}{N(0)}=1, ~~{D_{f_{q5}}(0)}=0,\\ \end{array}\right. \end{aligned}$$

(a15)

where $\Delta \varPhi (s),Q_1(s),F(s)$ and $F_q(s)$ are the Laplace transforms of $\Delta \varphi (t),~q_1(t),~f(t)$ and $f_q(t)$, respectively.

Denote the estimation error as $e_z=z-X.$ From (a15), it can be obtained that, when the closed-loop system reaches steady state, there is

$$\begin{aligned} e_{z_1}=\mathrm{O}(\dfrac{c_1}{{\bar{\omega }}_1^2}),~~e_{z_2}=\mathrm{O}(\dfrac{1}{{\bar{\omega }}_1^2}),~~e_{z_3}=e_{z_4}=e_{z_5}=0. \end{aligned}$$

The dynamic equation of the rigid mode can be rewritten as

$$\begin{aligned} \Delta \ddot{\varphi }= -k_p \Delta \varphi -k_d \Delta {\dot{\varphi }} +k_q \dot{q}_1 -b_{30}Ke_z. \end{aligned}$$

(a16)

Therefore, the steady-state error of the rigid mode $\Delta \varphi$ depends on $-e_{z_1}$.

Since ${\lim \limits _{t \rightarrow \infty }}\alpha _w,{\lim \limits _{t \rightarrow \infty }}M_{b_Z}$ and ${\lim \limits _{t \rightarrow \infty }}M_{q1}$ exist, (20) is obtained. $\square$